Craps Dice Roll Probability
Gambling games that use dice, such as the game of 'craps,' are often used to demonstrate the laws of probability. For two dice, the possible rolls and probability of each roll are usually represented by a matrix. Consequently, the SAS/IML language makes it easy to compute the probabilities of various events. The casino game of Craps is played with a set of two perfectly balanced six-sided dice with each die side having white dots numbering one through six. The game is played by tossing the dice from one of the short ends of the table to the other, making sure that both dice hit the wall on the opposite side of the table.
- Craps Dice Roll Probability
- Craps Dice Roll Probability Rules
- Craps Dice Roll Probability Rule
- Craps Dice Roll Probability Dice
The majority of casino games are known to offer either a low or a high house edge. Craps stands out from the crowd because it offers both. For one thing, this unique game features some of the best bets you can possibly make in a casino. For another, some of the more advanced, proposition bets in craps give the house a formidable edge over patrons that nearly reaches 17%!
Another distinctive trait of craps is that it is the only game of chance where players can actually bet something will not happen instead of backing the outcome they believe is most likely to occur, as is the case with Don’t Come and Don’t Pass bets.
These two features are what render this simple game of chance so unique and appealing in the eyes of millions of gamblers around the world.
As simple as craps seemingly is, you most definitely should take the time necessary to learn all the possible dice combinations along with their odds and probabilities before you invest any of your money in the game.
The purpose of this part of the guide is to introduce you to all dice combinations in craps and to help you make a distinction between true and casino odds. By the end, you will know whether dice control is effective in reducing the house edge and will be able to calculate your average expected losses at the craps tables.
Possible Dice Combinations in Craps
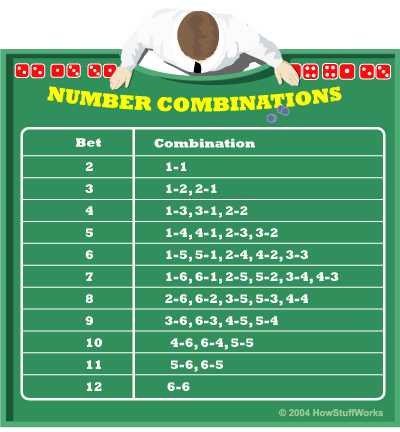
As we have previously explained in this guide, craps is a game of chance that plays with two six-sided dice, with each side having a different number of pips so as to represent numerical values 1 through 6. Each toss of the two dice can result in one of 11 possible numbers, namely numbers 2 through 12.
When two dice are in play, the number of possible dice combinations increases to 36 since each dice is practically a cube with six sides (6×6 = 36). Now, we want you to take a closer look at the table below and see whether you will be able to notice a trend.
| Dice Total | Number of Ways to Throw Total | Possible Combinations |
|---|---|---|
| 2 | 1 | 1-1 |
| 3 | 2 | 1-2, 2-1 |
| 4 | 3 | 1-3, 3-1, 2-2 |
| 5 | 4 | 1-4, 4-1, 2-3, 3-2 |
| 6 | 5 | 1-5, 5-1, 3-3, 2-4, 4-2 |
| 7 | 6 | 1-6, 6-1, 2-5, 5-2, 3-4, 4-3 |
| 8 | 5 | 2-6, 6-2, 5-3, 3-5, 4-4 |
| 9 | 4 | 3-6, 6-3, 4-5, 5-4 |
| 10 | 3 | 4-6, 6-4, 5-5 |
| 11 | 2 | 5-6, 6-5 |
| 12 | 1 | 6-6 |
You have probably noticed the column with the possible combinations is diamond-shaped. From this shape, it becomes apparent the number of combinations that may result in a toss of 7 is the highest, which automatically means the probability of this number being tossed is the highest.
As many as 6 combinations result in a 7 which is the main reason why the majority of seasoned craps players favor the Pass Line where a come-out roll wins when 7 or 11 are tossed, with a total of 8 possible winning combinations (six for a toss of 7 and two more for a toss of 11).
Do not be intimidated by the chart – there is one easy way for you to learn the combinations by heart. All you have to do is use 7 as a starting point and divide the remaining outcomes into groups of two. You can pair rolls of 6 and 8 since both outcomes have 5 possible combinations.
Next in line is the pair of 5 and 9, with each of these rolls having 4 possible combinations. The next pair comprises rolls of 4 and 10 with three dice combinations. Then you have 3 and 11 where the number of possible combinations drops with a unit to two.
The rolls of 2 and 12 are the easiest to memorize since there is only one possible dice combination for each of the two outcomes. Learning these combinations is essential because it helps you gain a better understanding of the odds and probabilities in craps. We tackle the subject in the next section.
Understanding the Odds and Probabilities of Craps Bets
This is the part most gamblers struggle with. Craps, like all other games of chance, is based on independent trials, which is to say the odds of the dice rolls remain constant and are not influenced by previous outcomes. This is easily the most important aspect of the game all craps players must understand. Yet, there are people who would invest their action in a given dice outcome simply because they have not seen it occur in a while.
Here it is important to draw a distinction between odds and probabilities. While interrelated, the two terms do not denote one and the same thing as many gamblers mistakenly presume. Probability is nothing more than the likelihood of an independent outcome occurring and is usually expressed either as a proper fraction or as a percentage.
To calculate the probability of rolling a certain value, you must know the number of dice combinations that result in it as well as the number of all possible dice combinations. For instance, the probability of tossing a 7 on the come-out roll is the highest at 16.67%. We can also say the probability is 6 in 36 because there are 6 winning combinations out of 36. This is called theoretical probability.
Odds, on the other hand, show you the ratio between winning and losing outcomes and are usually expressed as fractions. So for example, if you decide to make a Snake Eyes bet, which is a wager on a single roll of 2, the odds of winning will be 1 to 35 because there is only one winning combination for this roll while the remaining 35 combinations result in a loss. This corresponds to a theoretical probability of 2.78% because 1/36 = 0.02777 x 100 = 2.78%. Consult the table below to see the probabilities of rolling all two-dice totals in craps.
| Two-Dice Total | Probability as a Fraction | Probability of Rolling the Total as a Percentage |
|---|---|---|
| 2 | 1/36 | 2.78% |
| 3 | 2/36 | 5.55% |
| 4 | 3/36 | 8.33% |
| 5 | 4/36 | 11.11% |
| 6 | 5/36 | 13.89% |
| 7 | 6/36 | 16.67% |
| 8 | 5/36 | 13.89% |
| 9 | 4/36 | 11.11% |
| 10 | 3/36 | 8.33% |
| 11 | 2/36 | 5.55% |
| 12 | 1/36 | 2.78% |
The Difference between True Odds and Casino Odds
It is of essential importance for you to understand there is a distinction between true odds and casino odds. It is the discrepancy between the two that gives the house the edge that eventually grinds the bankrolls of imprudent gamblers down to nothing.
If you hang around a casino for an hour or so, you are more than likely to hear (losing) players complaining about the games being rigged as they exit the venue with empty pockets. These players are, in fact, correct to a certain extent. The games are indeed rigged against them but not because the casino resorts to cheating. Why cheat your patrons when there is a perfectly legal way of extracting their money?
Another Example
The main reason gamblers lose in the long term is the above-mentioned discrepancy between true odds and the odds the house pays you at when you win. The following example demonstrates how the house edge in craps works. Let’s presume you decide to make 36 consecutive Snake Eyes bets wagering a dollar on each. The mathematically correct odds for this bet are 35 to 1, which is to say 35 of all possible combinations result in a loss whereas only 1 combination (1-1) can lead to a win.
Now take a quick glance at the layout of the craps table. Can you see what it says? The payout for a one-roll Snake Eyes bet on 2 is 30 to 1 instead of being 35 to 1, as it should be. The same goes for a roll of 12 where again there is a single winning combination (6-6). This applies to all payouts in craps – they have all been reduced, making it possible for the house to extract consistent profits from its tables in the long term.
So you wager a dollar on Snake Eyes a total of 36 times in a row and the results are mathematically perfect, meaning that one of those 36 trials was indeed a winning one. Now, this is unlikely to happen in the short term but generally, the more you play, the closer your results get to the mathematical expectations for the game.
Players can easily figure out what the house edge is for any available bet in craps as long as they know its true odds, its payout, and its probability. Let’s demonstrate how this works for the Any 7 bet. This wager wins on a roll of 7, regardless of which one of its six combinations occurs. The payout for a winning Any 7 bet is 4 to 1. The probability is 6 in 36 possible dice combinations.
The calculations will run in the following manner: (6/36) x 4 – (30/36) = (0.166 x 4) – 0.833 = 0.666 – 0.833 = (-0.166) x 100 = -16.67. The figures in the first brackets correspond to the probability of winning with an Any 7 bet, which is then multiplied by the casino’s payout of 4 units per unit wagered. You then subtract the probability of losing (the figures in the second brackets stand for 30 ways to lose out of 36 possible combinations) from the result and get a house edge of 16.67% for the Any 7 bet.
As you can see, you will lose $16.67 (hence the “-”) per every $100 wagered on the Any 7 bet. In other words, your expected return with this bet will be in the negative at -$16.67. If you are still struggling to understand this, we suggest you go back to the Craps House Edge article of this guide where you will be able to find further explanations on the matter along with the true and casino odds of all available craps bets.

Distinguishing between “To” and “For” Odds
The trouble with most casual gamblers is that they are so engulfed in the action, they rarely pay any attention to anything else, including what’s in front of their eyes, right there on the table layout. If you take the time to carefully inspect craps layouts, you will undoubtedly notice there is something weird about the proposition bets section at some craps tables.
In some casinos, the layout states that you get 5 units “for” 1 unit wagered on Any 7 instead of the usual payout of 4 “to” 1. The same goes for proposition bets like the Hard 6 and the Hard 10 which pay 10 unit for each unit wagered instead of the usual 9 to 1. Many undiscerning gamblers are misled by this phrasing (that was the purpose in the first place) and are quick to assume their winning proposition bets return at enhanced odds. There is nothing of the kind, though.
The “for” payouts are basically the same since they include your initial stake. In contrast, the phrasing “to” distinguishes your net profits from your original bet. So in the case of the Any 7 bet, you get 4 units in net profits plus your original stake of 1 unit for a total of 5 units.
The wording of these payouts is no coincidence. Quite the contrary, the house uses this sly approach for the purpose of leading inexperienced craps players into believing they get higher payouts on the wagers with the steepest house edge when in reality, they are paid at standard casino odds. No matter what phrasing is used, we would like to remind you not to waste your money on proposition bets. The monstrous house edge you combat with these wagers is not worth it even if the payouts were indeed “enhanced”.
Figuring Out Your Expected Loss in Craps
This section is somewhat a continuation of the True Odds one. You are probably scratching your head wondering why on earth would we teach you how to calculate your expected losses. What you want to know is how to determine your hourly expected profits, right? We hate to break this to you but craps is a negative expectation game which is to say your expected value in the long run will always be in the negative due to the house edge, i.e. you will inevitably lose money to the casino in the long term.

It is important for you to learn to calculate your expected loss so that you know at what average rates you will lose money per hour. This depends on your action, the types of bets you make, and their house edge. The formula is quite simple – you must multiply the number of rounds you play per hour by the amount you wager, the house edge, and the number of hours you intend to play.
So, let’s suppose you play at a slower pace and go through 160 rolls, betting $5 on Any 7 for one hour. The calculations will look like this: 160 x $5 x 0.166 x 1 = $133 on average. This is the average rate at which you lose money with Any 7 wagers but you arrive at this amount after a gazillion of independent trials. In the short term, you might end up losing much more or far less within an hour. The bottom line is the longer you play, the closer you get to these expected loss figures.
Can Dice Control Influence the Probability of Rolling Certain Combinations?
Some craps players would attempt to influence the outcome of the rolls by using an advanced technique, called dice setting or dice control. The main idea here is that you can skew the odds in your favor by tossing the dice in a specific manner. To achieve this, the shooter must toss the dice at the correct angle in order to allow them to produce the desired outcome.
The toss itself should be performed with as little hand movements as possible so that the dice do not tumble as much before they hit the back wall of the craps table. The dice must be picked in a particular way as well. The shooter’s wrist remains locked during the toss, i.e. there is no twisting motion when the dice are thrown. It makes sense this technique takes ages to master but the real question is does it really help you influence the outcome of the roll?
Renowned gambling author Stanford Wong is among the proponents of the efficiency of this technique and wrote extensively about it in his book Wong on Craps. The subject was also tackled by author Christopher Pawlicki in his 2002 book Get the Edge at Craps: How to Control the Dice. Some mathematicians and gambling experts are of the opinion the technique either does not work or is impossible to execute successfully in the casino environment.
Others are willing to give dice control some credence arguing that it might possibly work, provided that the dice do not hit the back wall of the craps table. Unfortunately, most casinos require the dice to hit the back wall in order for the roll to be considered valid. The bottom line is most members of the advantage play community still distrust the concept of overcoming the house edge in craps through dice control.
Chart on the possibility of each number being rolled.
When playing online Craps, it is important to understand the probability of each dice combination being rolled. Once you know what the probability is, you can work out what the house edge is on particular bets. The odds paid to you, the probability of your bet being successful and the house edge are all important factors in how lucrative Craps will be for you.
Why Use a Craps Probability Chart?
The more information that you have at our disposal, the better decisions you can make. A more informed and smarter Craps player has a much better chance of winning on a regular basis. Whether you are a seasoned online craps professional or a casual players, a craps probability chart is crucial to understanding the probability of each dice roll result. With this knowledge, you can make smarter bets at the craps table.
For example, you can see on our craps probability chart that the most common number to come up after a dice roll is a seven. This is the reason that most craps professionals will recommend the pass line as the smartest bet you can make when formulating a solid craps strategy. On the other hand, you can easily see that, according to our craps probability chart, the most uncommon dice roll results are two (or snake eyes in many circles) and 12. Taking this into account, making a bet on two or 12 would likely not be the best bet that you could make.
How to Use a Craps Probability Chart
Obviously, there is nothing to stop you from printing out our craps probability chart and posting it by your computer any time that you are playing craps online. However, by reading our extensive section on the rules and etiquette of craps in offline casinos, you will also find that there are no rules preventing you from printing out the craps probability chart (or copying it on paper with a pencil) and bringing it with you to your favorite offline craps casino.
Every time you are considering a bet, you can consult the craps probability chart to see if the odds match up to the amount of your bet. However, a craps probability chart is simply a tool and cannot alone formulate your entire craps strategy. Some situations require a great deal more information than a craps probability chart before determining whether or not the bet is wise. For more information, you can consult our craps strategy section if you are unsure how to discover the total odds of a bet being successful. For now, memorizing the craps probability chart is your first step towards becoming a craps professional.
The game of craps is a wonderful opportunity to enjoy an energetic casino experience. The high enthusiasm for the game is mixed with the lure of “tempting fate” with the chance of betting on dice rolls. Players and onlookers rejoice together when the shooter hits the point and satisfies several bets, or lament together when the “dice” are against them.
In addition to this, part of what craps offers interested gamblers is a simple way to work the odds of the casino to a player’s favor. Winning at craps is entirely about learning the true probabilities of certain dice rolls occurring and making sure a player has money on the rolls with the best probability. The surest way to win in craps is to maximize bets with good odds and avoid bets with very poor odds.
Dice Probability
The essential starting ground for craps odds and probabilities is with the dice roll itself. Since bets are made based on the potential outcome of the dice, knowing how often, or how infrequently a number combination will occur is the foundation for betting odds in craps.
A pair of standard six-sided dice offers thirty-six possible number combinations that can occur with each roll. First, players need to understand that craps, and dice probabilities are literally roll by roll. Mathematically, what makes craps a game of chance is that every time the shooter picks up the dice, the exact same overall odds apply. There are not cumulative effects roll by roll, so the gambler’s fallacy of “what hasn’t occurred in a while is more likely to occur soon” cannot apply to craps, though many players bet like it will.
In reality, the dice odds stack in a pyramid that symmetrically pairs combinations of numbers, which have the same odds of occurring. The most frequently rolled number is a seven, which a player can mathematically expect to see once in every six rolls. This high probability is due to the fact that seven has the highest possible number of dice combinations to form it between the two dice.
The craps dice odds and probabilities list as follows:
- 2 and 12 have only one way they can be formed on two dice, thus carrying odds of 35 to 1 (a one in thirty-six chance of being rolled).
- 3 and 11 have two possible formations, so the odds of these appearing are 17 to 1.
- 4 and 10 each have three potential combinations, improving the odds of showing either of these to 11 to 1.
- 5 and 9 have four possible formations each, thus holding odds of 8 to 1.
- 6 and 8 are the second most frequently rolled combinations, with five possible ways to see a six or eight on the dice. The odds of either are 6.2 to 1.
- 7 has six possible formations, the most possible and gives it 5 to 1 odds of showing.
These odds are calculated by taking the number of possible formations out of thirty-six, reducing it to it’s lowest denominator and placing it in odds terms. For example, the four has three ways of being formed on the dice, thus a 3 in 36 chance of occurring or, reduced a one in twelve chance of being rolled. In odds terms one in twelve becomes 11 to 1. Understanding and remembering the dice odds is the basis for effective betting in craps.
The chance factor comes in with the fact that even though these odds are mathematically true over dozens and hundreds of rolls, and can be trusted to show in these ratios over a larger sample, they won’t “perfectly” occur with human shooters. Thus a shooter who gains six rolls can sometimes roll all six without showing a seven. This is what brings the element of risk and excitement to craps betting.
Making Good Odds Bets
There are three groupings of bets that have the best odds and the lowest house edge that a craps player can come by. These are the bread and butter of a craps player’s existence, and the wisest and winningest craps players use these bets like clockwork to maximize their odds of taking home cash, not just laying it down on the table. These are the most commonly used bets, as well.
On the right bettor side are the pass bet and the come bet. Pass bets are made during the come out roll; come bets are made after the shooter has established the point. Both of these are sweeping bets that the shooter will roll seven or eleven or a point number before rolling craps, 2, 3 and 12, the low frequency numbers. Pass bets and come bets parallel each other. Both of these bets have true odds of 251 to 244. The house edge, or casino advantage on these bets is one of the lowest at 1.41 %, which means bettors placing pass or come bets have a very good chance of winning this bet.
The wrong bettor partners to the pass and come bets are the don’t pass and don’t come bets. These bets go against the shooter (and thus are sometimes disliked by players), betting that the shooter will hit craps before hitting his point or seven. The house edge on these wrong bets actually drops further to 1.36%, and the odds of these bets showing are 976 to 949.
Free Odds Bets
The free odds bets that go with these are even better from an odds standpoint. The best bets to make in craps, free odds bets multiply a pass or come bet (or a don’t pass or don’t come bet). The advantage of these is the house edge gets lowered to almost zero, meaning players have a good chance of winning these bets at their actual mathematical odds. The odds bets have to be made in conjunction with the main pass/come and don’t pass/don’t come bets. On the right bettor side for pass and come odds bets, making this bet is called “taking the odds.” On the wrong bettor side, odds bets with don’t pass and don’t come bets are called “laying the odds.”
The pass odds and come odds bets have actual odds 2 to 1 on four and ten, 3 to 2 on five and nine, and 6 to 5 on six or eight. The payout ratios for these odds bets match the odds ratios for each number. On the don’t pass/don’t come odds side, the true odds are 1 to 2 against four and ten, 2 to 3 against five and nine, and 5 to 6 against six and eight. Again, the payout ratio when one of these bets wins is the same as the odds of occurrence.
Place Bet Odds
The third category of bets that use the dice probabilities to a great advantage are the place bets on the box numbers at the top of the craps table. Essentially, a place bet makes the same assertion as the free odds bet, that the shooter will roll one of these numbers before a seven or craps. The advantage to place bets is they can be made independently and don’t require a pass line bet first. However, the disadvantage is they payout at poorer odds than the free odds bets.
Four of the six possible place bets have a low house edge, making them still some of the better bets to make as far as player advantage. Place bets on six and eight have the third lowest house edge at 1.52%, making them very profitable bets. The odds of winning on place six and place eight bets are 6 to 5 or 45.45%. Payouts are at 7 to 6 odds. For even dollar payouts, player should bet in increments of $6 on these place bets, preventing rounding down by the casino on paying winnings.
Place bets on five and nine are also still lower house edges than most bets on the table at 4%. Players have a 40% chance of winning or 3 to 2 odds, and they payout at 7 to 5 odds. Place these bets in multiples of $5 for even dollar payouts without rounding.
Place bets on four and ten are still decent bets, but the house edge starts to climb–it’s 6.67%. The odds of winning a place four/place ten bet are 33.33% but if it does win, the payout rate is 9 to 5. These bets should also be made in $5 increments for even payout amounts.
Another advantage to place bets is that when a place bet wins, the dealer pays the player’s winnings but leaves the original bet on the table effectively starting another place bet. The player doesn’t have to add money to the bet to continue it. Players can ask the dealer to “turn off” the standing place bet or remove it if they wish.
A few online casinos offer the wrong side version of place bets called place bets to lose. Live casino Star City in Sydney, Australia also allows place bets to lose. These bets flip the bet to lose when the shooter hits the number first, but win if the shooter rolls a seven before the number. The odds of winning these place bets go from 54.55% to 66.67%, rather than under 50% for their right side counterparts. Place to lose on six and eight pay out at 4 to 5; on five and nine they pay out at 5 to 8; and on four and ten they pay out at 5 to 11.
Craps Dice Roll Probability
Betting the Field Odds
The field box bets are right in front of the players and can be enticing bets. Their major disadvantage stands in that they are single roll bets, only valid for the next immediate dice roll. The house edge at 5.56% is smaller than many other craps bets, but still starting to be larger than is wise for a player to use often. The payout odd for field bets on three, four, nine, ten and eleven are 1 to 1, even money. For the more rarely rolled two and twelve, the payout odds are 2 to 1. The true odds of betting on the field are 5 to 4.
Odds on Proposition Bets
The proposition bets located in the center of the craps table actually hold the poorest odds for a player, and with high house edges hold great odds for the casino winning. Craps experts consistently advise staying away from these various “sucker bets” that catch novices or people who have not bothered to learn a few key points about playing craps.
Proposition bets are mostly single, next roll bets on specific numbers. The rates of the occurrence for the dice roll is the first basis for the enticingly high payouts, but the house edge factors in on the lesser likelihood that the single next roll will produce that number or combination of numbers.
The list of odds for the main proposition bets is as follows:
Craps Dice Roll Probability Rules
- Craps 2 or 12 has true odds of 35 to 1, that single dice combination. The payout odds are 30 to 1, with a huge house edge of 13.89%.
- Single number bets on 3 or Yo (11) carry true odds of 17 to 1, but the casino pays 15 to 1 odds on a winning bet. The house edge is 11.11%
- A Hi-Lo bet matches the odds of 3/11 bets with true odds of 17 to 1 and payout odds of 15 to 1. The house edge is also 11.11%.
- Any Craps bets (looking for 2, 3, or 12) have true odds of 8 to 1, payouts of 7 to 1 and that ever-large 11.11% house edge.
- C-E or Craps-Eleven bets have true odds of 5 to 1. The payouts vary at 3 to 1 for craps (2, 3, 12) and 7 to 1 on eleven. This also carries an 11.11% house edge.
- Any 7 bets have true odds of 5 to 1 with payout odds at 4 to 1. Any 7 has the largest house edge on the table at 16.67%.
- Horn bets carry a higher house edge of 12.5%. The true odds are 5 to 1. Payout odds are 27 to 4 on two or twelve and 3 to 1 on three or eleven.
World or whirl bets are a variation of the horn bet on 2, 3, 7, 11 and 12. The true odds are 2 to 1. The payout odds on two or twelve are 26 to 5 and on three or eleven are 11 to 5. The house edge is 13.33%.
Big 6 and Big 8
The corner proposition bets of Big 6 and Big 8 have been banned from Atlantic City casino craps tables because the odds make them such poor bets. With a large house edge of 9.09%, bets on Big 6 and Big 8 only payout even money, though the true odds of rolling a six or eight before a seven, like in Place 6/Place 8 bets is 6 to 5.
Hardways Bets
Hardways proposition bets can be ongoing bets, but these are also costly bets to make. The hard four and hard ten bets have an 11.11% house edge and pay 7 to 1 if they win, but only carry an 11.11% chance of winning. Hard six and hard eight bets payout at 9 to 1, but only have a 9.09% chance of winning. The house edge for those two bets is 9.09%. It doesn’t seem like a bet bodes well for a player when the odds of winning equal the odds of the house advantage over the player.
Buy Bets and Lay Bets
For players who like to use place bets but who don’t like the odds, the option exists to buy the points for a 5% house commission that reduces the house advantage to “fair odds.” Some casinos require that commission up front; others take the commission on winnings. Most players will advise staying away from Buy and Lay bets. Paying extra to level the odds is simply not worth it. However, some players do like to use these bets.
Buy bets have a standard 4.76% house edge on all three pairs of bets. The odds are:
Craps Dice Roll Probability Rule
- Buy four and Buy ten have 2 to 1 odds.
- Buy five and Buy nine have 3 to 2 odds.
- Buy six and Buy eight have 6 to 5 odds.
Craps Dice Roll Probability Dice
Lay Bets are the “Place to Lose” or wrong side lay odds commission bets. The lay bet odds are slightly different than the buy bet odds.
- Lay four and Lay ten have 1 to 2 odds with a house edge of 2.44%.
- Lay five and Lay nine carry odds of 2 to 3 and a house edge of 3.23%.
- Lay six and Lay eight have 5 to 6 odds and a 4% house edge.
Overall Craps Odds
Some players ask about their overall chances of winning or losing at craps. Honestly, with most games of chance players will do well to expect to lose more than what they win. Statistically, in a particular session the mathematical odds reinforce the odds of losing are slightly greater than the odds of winning.
The odds of breaking even are about 0.67%.
For a truly big picture perspective on the odds of playing craps, probabilities calculated from simulating 1,000,000 sessions of 100 come out rolls each leave a players odds of winning and losing overall at the following percentages:
- Winning $1 to $25: probability 28.64%
Losing $1 to $25: probability 30.06% - Winning $26 to $50: probability 14.43%
Losing $26 to $50: probability 16.36% - Winning $51 to $75: probability 3.91%
Losing $51 to $75: probability 4.64% - Winning $76 to $100: probability 0.564%
Losing $76 to $100: probability 0.65% - Winning over $100: probability 0.0418%
Losing over $100: probability 0.0422%
With so many calculations for craps odds and probabilities, the best way for a player to utilize this information is to remember the dice probabilities and the most beneficial bets to use. Craps tables list the payout odds for the higher bets as part of inviting players to spend money on bets that the house is more likely to win than the player. Seeing a 30 to 1 payout option makes some folks eager to bet there for the big return, but the savvy player will remember that the odds of that single two or twelve showing up on the next roll are 35 to 1. There are many more useful places to invest those bets. Strong players will use these odds and probabilities on craps to make guided decisions about where best to bet their hard earned money.